Magnets are ubiquitous in modern life, used in motors, generators, wind turbines, steel foundries, toys, and all sorts of technological equipment. They are also essential for several distinct classes of device, including particle accelerators.
Particle Accelerator Magnets
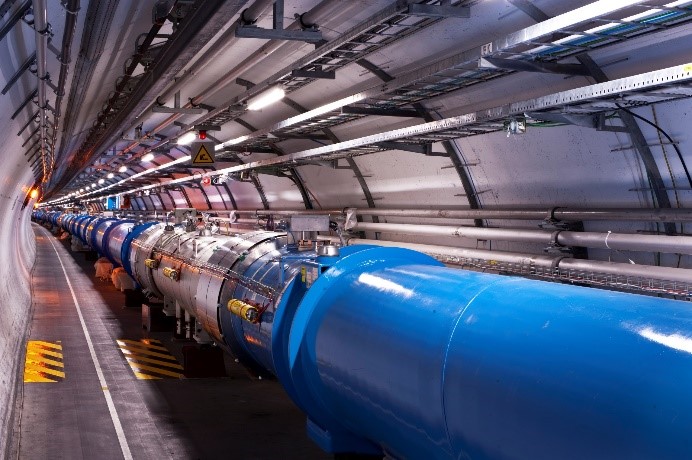
Beams from particle accelerators are used to treat cancer patients and to create radioactive isotopes for medical diagnostics, medicine and sterilization of instruments. They are used to make computer chips and to deal with biological waste. Particle accelerators are also used to study proteins and molecules, including viruses such as COVID-19. The most powerful particle accelerators – such as the LHC at CERN (see Figure 1) – are used for particle physics, built by multi-national collaborations seeking to shed light on the basic constituents of the universe.
Particle accelerators come in several different forms – linear accelerators or linacs, and circular accelerators, with cyclotrons and synchrotrons being the most common. They all use radio frequency electromagnetic waves to accelerate charged particles, and various kinds of magnets – permanent, resistive and superconducting – to bend and focus the beams of particles.
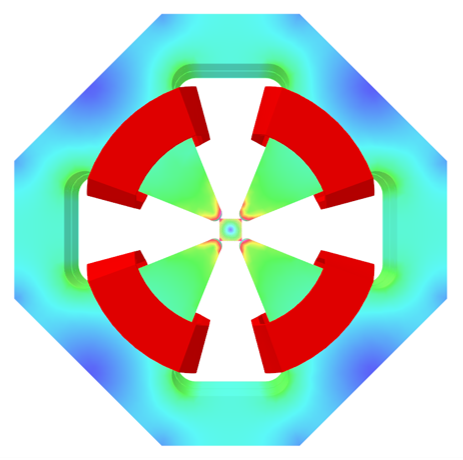
The magnets used to control the beam must be designed accurately if the beams are going to remain focused and reach the target at the desired point. SIMULIA Opera is used to support the design of different types of magnets, with each performing a specific role in these ground-breaking devices. Figure 2 shows a quadrupole magnet modelled with SIMULIA Opera, with the magnetic flux density displayed on the steel poles, and also on a cross-section where the beam would pass.
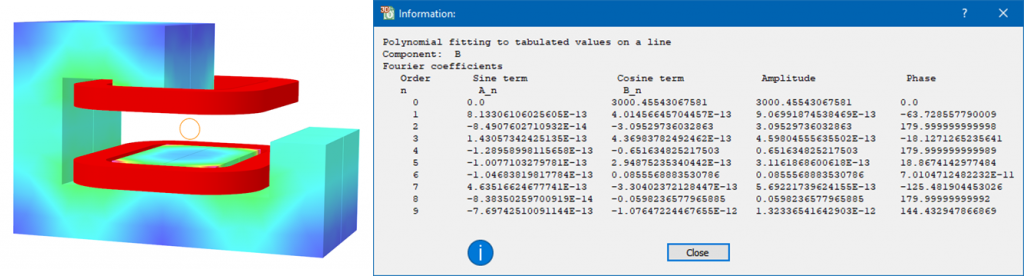
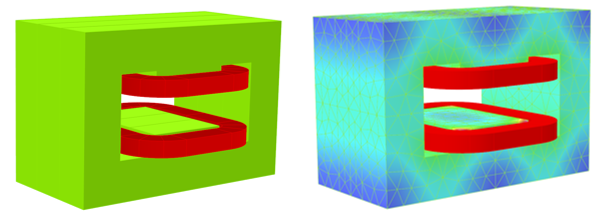
While particle accelerators can take different forms, in each there will commonly be bending magnets, focusing magnets, correctors, and kickers. Typical design considerations include desired outcomes, such as flux density and homogeneity, while observing constraints such as induced forces, heat fluxes and remaining in the desired operating parameters of available materials. Figure 3 shows a ¾ display of a dipole model, as well as the calculation of Fourier coefficients of the flux density on a circle around the beam region – the usual method to show field homogeneity.
Bending magnets, as their name suggests, use the forces exerted on the particle due its movement in a magnetic field to steer the beam. Force is calculated from the Lorentz force law:
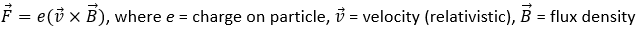
A 3D dipole model is shown in Figure 4.
Focusing magnets use field gradients to focus the beam into its desired shape and size. The shape of the poles creates linear field gradients in the beam region:
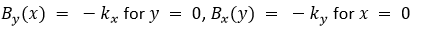
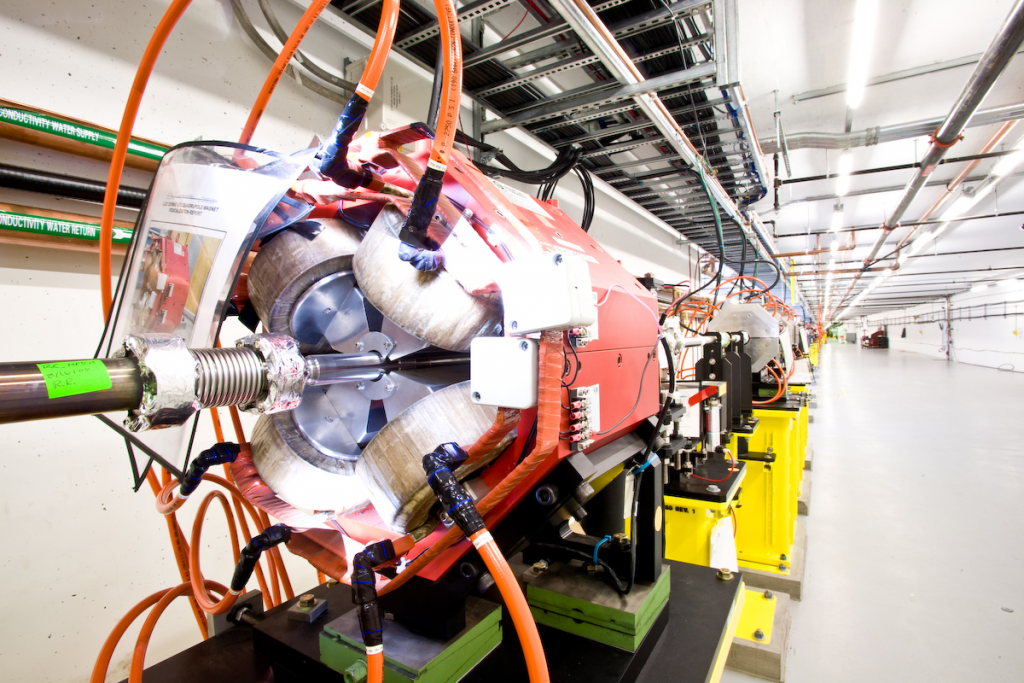
Figure 5 shows a quadrupole magnet from the SLAC National Accelerator Laboratory.
Other imperfections in the beam (such as it becoming unstable over time) arise due to magnet imperfections, misalignments or intra-beam effects for instance, can be addressed using higher order multipole magnets, supplementing the dipoles and quadrupoles commonly used for steering and focusing. These types of magnets might be DC, but they could also be pulsed or programmable.
Kicker magnets are pulsed rapidly, redirecting the beam to a new trajectory. A very important design characteristic of a kicker magnet is how quickly it can be turned on and off – after all, the beam in the 27 km circumference LHC goes round about 11,000 times each second!
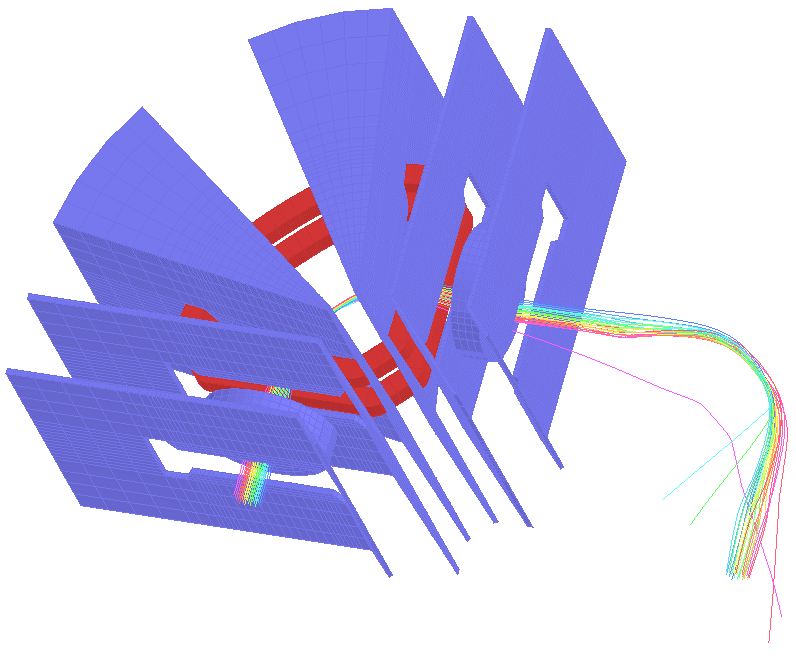
Charged particle beams can be simulated in Opera, and tracked through the complex fields generated by these magnets. Figure 6 shows charged particles being tracked through a specialized dipole called a fragment separator, used to identify short lived radioactive isotopes.
Due to the ever-higher magnetic fields required to advance this science, superconducting magnets are often used, which exploit the behaviour of superconductors, at very low temperatures (around 4 K), to conduct extremely high electrical currents with zero resistance. Superconducting dipole coils modelled in SIMULIA Opera are shown in Figure 7.
Particle accelerators are continually pushing the boundaries of science and medicine. The only way to design and build these devices with confidence is by using simulation to predict their performance, both during normal service and during potential operational faults such as a quench of the magnet – where a superconducting magnet starts to warm above its operating temperature and dramatically returns to its normal conducting state. SIMULIA Opera is a vital tool allowing engineers confidence that their designs will operate as expected.
Want to learn more about Opera and connect with other Opera users? Visit our SIMULIA Community to discover the latest tips, to ask questions and to share your expertise. Our dedicated Opera Learning Resources page is a great place to start exploring.
SIMULIA offers an advanced simulation product portfolio, including Abaqus, Isight, fe-safe, Tosca, Simpoe-Mold, SIMPACK, CST Studio Suite, XFlow, PowerFLOW and more. The SIMULIA Community is the place to find the latest resources for SIMULIA software and to collaborate with other users. The key that unlocks the door of innovative thinking and knowledge building, the SIMULIA Community provides you with the tools you need to expand your knowledge, whenever and wherever.
Dr Ben Pine has worked as an Industry Process Consultant for Dassault Systèmes for 2 years. Before that, he was an Accelerator Physicist at Rutherford Appleton Laboratory for 15 years where he worked to study, operate and upgrade the ISIS Spallation Neutron Source proton synchrotron. His main area of research was high intensity transverse beam dynamics including the effects of space charge. Ben got his DPhil in particle physics from the University of Oxford, studying part-time while at the laboratory. At Dassault Systèmes, Ben works with the Opera team, spending most of his time on accelerator and MRI magnets, magnetization and quench.